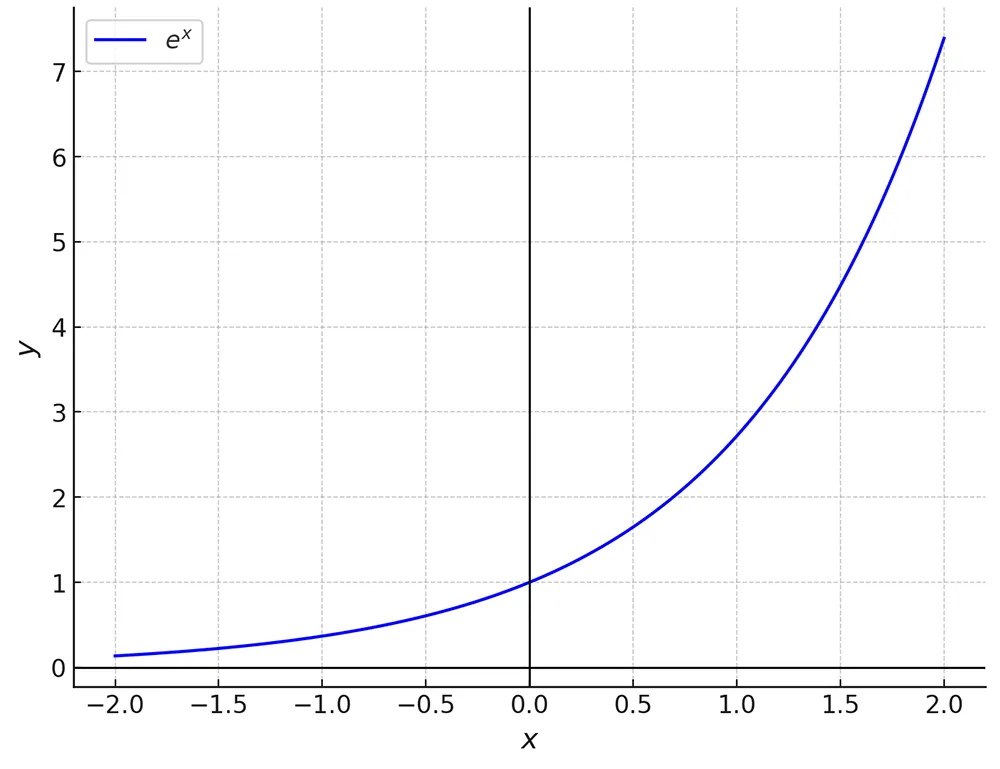
Derivative of Exponential

In order to find the derivative of \(e^x\), we can use the limit definition of the derivative:

\[ \frac{d}{dx}e^x = \lim_{h \to 0} \frac{e^{x+h} – e^x}{h} \]

\[ \Rightarrow \frac{d}{dx}e^x = \lim_{h \to 0} \frac{e^x (e^h – 1)}{h} \]

Since \(e^x\) is independent of \(h\), we can factor it out of the limit:

\[ \Rightarrow \frac{d}{dx}e^x = e^x \lim_{h \to 0} \frac{e^h – 1}{h} ~~~~~ (1) \]

We use the Taylor series expansion of \(e^h\):

\[ e^h = 1 + h + \frac{h^2}{2!} + \frac{h^3}{3!} + \dots \]

\[ \Rightarrow e^h – 1 = h + \frac{h^2}{2!} + \frac{h^3}{3!} + \dots \]

\[ \Rightarrow \frac{e^h – 1}{h} = 1 + \frac{h}{2!} + \frac{h^2}{3!} + \dots \]

As \(h \to 0\), all terms involving higher powers of \(h, h^2, h^3 …\) tend to zero

\[ \Rightarrow \lim_{h \to 0} \frac{e^h – 1}{h} = 1 \]

Thus, (1) becomes:

\[ \frac{d}{dx}e^x = e^x \]